| Home » Articles » LESSON NOTES (Mathematics) » Lower Secondary |
|
Quadratic Expression and Equation introduction In this part we will explain the meaning of Quadratic expression and equation so as to make you bright understanding on next lesson: Before we continue, let’s check the meaning of expression. In mathematics we have something called term; a term is a single number or letter that expresses something. For example. 5, 2x, 1.9, 1/2, etc. all these are terms. When we join these terms with any operation symbols like +, - , ×, and ÷ we get mathematical expression. Now what is Quadratic? Quadratic deals with variable with highest power "2”. So in quadratic expression we define as the expression which its highest power of the variable is "2”.
Example: x2+ 4, 4m2 + m – 5, r + 5 – 6r2 etc. all these are called quadratic expression. Note that all must have variable with highest power of 2. If any variable found with power greater than 2 such as x3 or n4 and something like that; that won’t be quadratic expression.
Quadratic equation: The only thing that makes an expression to be an equation is an equal sign "=”; if we add an equal sign in any mathematical expression, we’ll change it to equation. For example: 2 + 4, this is an expression but if we add equal sign it will be an equation "2 + 4 = 6”. Even this quadratic expression x2 + 4, but if we add equal sign it will be an equation "x2 + 4 = 0”.
Note: after equation it is useful to write the value next to equal sign as we did on those examples.
So in quadratic equation we define as the equation which its highest power of the variable is "2”.
EXAMPLES: Quadratic Expressions: (1) x2 + 5x (2) v2 – 2vn + n2 (3) m – 6 + m2 (4) x2 – b2 (5) 6y + y2 + 9
Quadratic Equation: (1) x2 + 5x = 4 (2) v2 – 2vn + n2 = 0 (3) m – 6 + m2 = 0 (4) x2 – b2 = 2 (5) 6y + y2 + 9 = 0
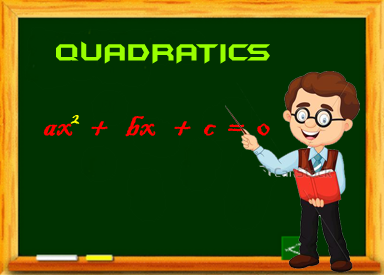
The formula of quadratic is formed as: ax2 + bx + c where "a” is coefficient of x2, "b” is the coefficient of x and "c” is constant term. For more about coefficient make revision by clicking here. This is the first sub topic of this topic.
Click here to continue with next tutorial about Operation in Quadratic Expression
********************************* ©Yahyou M.
| |
| Views: 3305 | |
| Total comments: 0 | |